Alumni

Bio
Growing up in Calgary, Paul-Jean Letourneau avidly pursued the arts almost exclusively, doing a lot of drawing, acting, and some music. Some of his artistic pursuits included writing comics, acting in and designing playbills for productions, singing in a rock band and in the tenor section of the school choir, and doing some short films.
Around the age of 16, Paul-Jean underwent a phase transition of sorts and became interested in mathematics, to bring him closer to things like fractal geometry. While still dabbling in artistic endeavors, he devoted himself to learning the sciences, and physics in particular. In 1998 Paul-Jean enrolled in the Honors Physics program at the University of British Columbia (UBC).
While in the Physics program at UBC, Paul-Jean did a number of work-experience placements, including Medical Imaging at the Vancouver General Hospital, Protein Nuclear Magnetic Resonance (NMR) at the University of Alberta, Geophysics in Calgary, and Biophysics at the National Institutes of Health in Bethesda, Maryland. He graduated with a B.Sc. in Physics in December 2003, and is currently working in the laboratory of Dr. Brian Sykes at the University of Alberta (a former co-op employer), solving for the 3-dimensional structure of a large muscle protein called troponin C, using NMR. He will enter graduate school in September 2004.
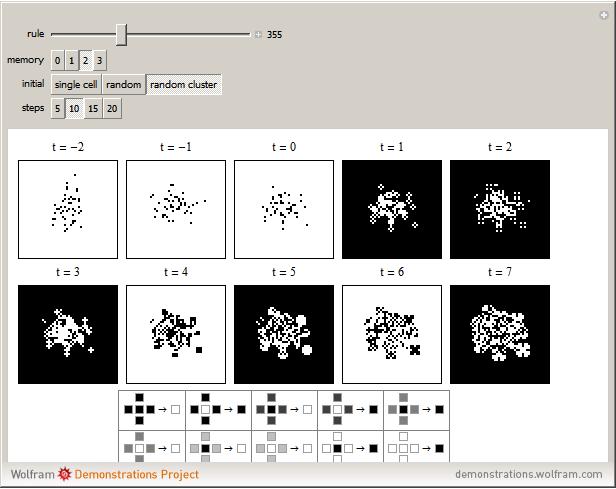
Project: Memory Effects in Cellular Automata
In the live experiment with Stephen Wolfram, we investigated the phenomenology of the elementary rules with one of the cells in the local neighborhood shifted a certain number of steps in the spatial dimension. For my project, I looked at what occurs when one of the cells in the neighborhood is shifted in time instead of in space. The neighborhood of cell i at time step t in an elementary rule is normally taken to be cells i-1, i, and i+1 of time step t-1. In the time-shifted case, certain of the cells in the neighborhood would be shifted more than one step back in time. For example, this time-shifted neighborhood might consist of cells i-1 and i+1 at time step t-1, and cell i at time step t–p, where p is the characteristic time shift.
Project-Related Demonstrations
Outer Totalistic 2D Cellular Automata with Memory
Favorite Two-Color, Radius-2 Rule
Rule chosen: 1464708970
My favorite r=k=2 rule is rule number 1464708970. This isn’t a very “sexy” rule from a visual point of view, but I think it has the potential to support structures similar to ones I recently found in rule 146. This rule was an interesting result from a search I did for structures similar to the “monoliths” I saw in rule 146. The basic idea was to look for rules that supported structures that appeared “suddenly,” in the sense that they have a characteristic size that is larger by some factor than the typical structures in rows immediately before it. In other words, I wanted to find rules with structures that sort of “jumped out of the background.” The fancy term I like to use is “emergent,” with deference to some kind of condensed matter physics terminology (or at least my own obscure usage of it!).